Circuit Analysis 2

Learning Objectives Superposition Thévenin’s Theorem Norton’s Theorem Maximum Power Transfer Theorem Quiz Useful Equations

Maximum Power Transfer Theorem

Set the real and imaginary values of the impedances as well as the magnitude and phase of the voltage and current sources for each network to find the load impedance for maximum power to the load.

\(Z_1=\) \(+j\) \(\Omega\)

\(Z_2=\) \(+j\) \(\Omega\)

\(Z_3=\) \(+j\) \(\Omega\)

\(E_1=\) \(\angle\) \(^\circ \text{V}\)

\(I_1=\) \(\angle\) \(^\circ \text{A}\)

\( Z_{Th} = (Z_1||Z_2)+Z_3 = \) Ω
\( E_{Th} = \frac{Z_2}{Z_1+Z_2}E_1 = \) V

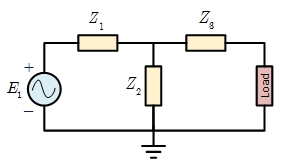
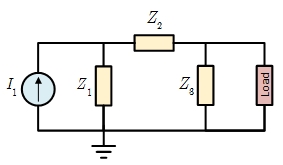
Maximum Power delivered to the load:

\( P_{Lmax} = \frac{E_{Th}^2}{4R_{Th}} = \) W

Maximum Power delivered by the source:

\( P_{Smax} = 2 P_{Lmax} = \) W

\( Z_N = (Z_1+Z_2)||Z_3 = \) Ω
\( I_N = \frac{Z_1}{Z_1+Z_2}I_1 = \) A

Maximum Power delivered to the load:

\( P_{Lmax} = \frac{I_N^2R_N}{4} = \) W

Maximum Power delivered by the source:

\( P_{Smax} = 2 P_{Lmax} = \) W

Module 5: Network theorems

Maximum Power Transfer Theorem