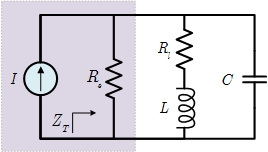
● Series resonant frequency:
\( f_s = \frac{1}{2\pi \sqrt{LC} } = \)?Hz
● Parallel resonant frequency:
\( f_p = f_s\sqrt{1-\frac{R_l^2C}{L}} = \)?Hz
● Maximum impedance frequency:
\( f_m = f_s\sqrt{1-\frac{R_l^2C}{4L}} = \)?Hz
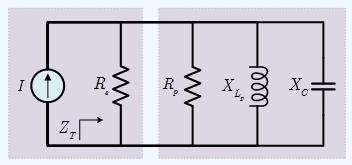
Parallel network equivalent at the terminals for the series R-L branch:
● \( X_L = 2\pi f_pL = \)?Ω
● \( R_p = \frac{R_l^2+X_L^2}{R_l} = \)?Ω
● \( X_{L_p} = \frac{R_l^2+X_L^2}{X_l} = \)?Ω
● Total Impedance:
\( Z_{T_p} = R_s || R_p = \)?Ω
● Maximum Impedance:
\( X_L(f_m) = 2\pi f_m L = \) ?Ω
\( X_C(f_m) = \frac{1}{2\pi f_m C} = \) ?Ω
\( Z_{T_m} = R_s || \sqrt{R_l^2+X_L(f_m)} || X_C(f_m) = \)?Ω
Coil Quality Factor:
\( Q_l = \frac{2\pi f_pL}{R_l} = \)?
Quality Factor:
\( Q_p = \frac{Z_{T_p}}{X_{L_p}} = \)?
Bandwidth:
\( BW = \frac{f_p}{Q_p} = \)?Hz
Half Power Corner Frequencies:
\( f_1 = f_p - \frac{BW}{2} = \)?Hz
\( f_2 = f_p + \frac{BW}{2} = \)?Hz